Polyhedra and hexagons

[Questo post è in inglese. Leggi qui la versione italiana]

The most well-known polyhedra, fo sure, are the regular ones, also called platonic: tetrahedra, octahedra, cubes, icosahedra, and dodecahedrons.

They are made of triangles, squares and pentagons... But can we make one out of hexagons?

The answer is no, at least if we want them to be equal and regular. This follows from the fact that putting three hexagons side by side, takes up the whole 360° angle (120° each one), and we can't bend them to make something three-dimensional.

The triangles take up the whole angle too, but we need six of them to do it. So, with only three, four or five we can create polyhedra: the tetrahedron, the octahedron and the icosahedron respectively.

What if we settle to only have some faces that are hexagons? In this case, we can construct polyhedra. For example, using pentagons, we can make a truncated icosahedron, which is the football's ball.

But let's suppose that we want all the faces to be hexagons.

Maybe it suffices to take them not regular? For example, there exists only one polyhedron made of squares (that is, regular quadrilaterals): the cube; but, if we don't ask them to be regular, there are others, one of them is the rhombic icosahedron. Then, maybe, there are no polyhedra made of regular hexagons, but there are made of irregular ones.

The answer, sadly, is always no!

Using Euler's formula for polyhedra, that says

vertices + faces = edges + 2 ,

(that is, the number of vertices, added to the number of faces, is equal to the number of edges plus two) one can prove that you can't construct a polyhedron made only of hexagons, it doesn't matter if regular or not!

This is very sad news for polyhedra and hexagons lovers :(

But don't worry! Nothing is over!

Euler's formula is only true for polyhedra that are "balls", which means that they have no holes. But Euler's formula for donut-polyhedra is different:

vertices + faces = edges .

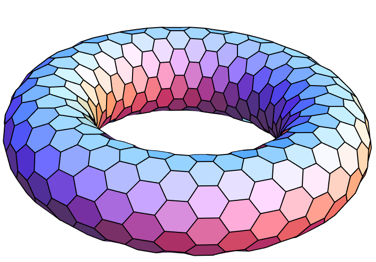
Thus, we can present a polyhedron made only of hexagons! Ladys and gentelmans... the Szilassi's polyhedron!

It has seven faces, all hexagonal. Each face has six edges and thus touches all the other faces. Six of those faces are concave hexagons, equal in pairs. The last one is a convex hexagon, the only one, and it is "in the hole".

This is its net:

Unfortunately, someone may say that concave hexagons are ugly.

I disagree. But, for those people, we'll search if it is possible to have a polyhedron made only of convex hexagons.

Searching on the internet, one can find this picture: a donut polyhedron exactly as we search it.

But... there is something wrong. This polyhedron can't exist! This is why:

We say that our polyhedron has n hexagonal faces.

Then, its edges are 3n, because every face has 6 edges, and every edge belongs to two faces.

Take now Euler's formula for donut polyhedra: vertices + faces = edges, then, soubstituting faces = n and edges = 3n, we obtain vertices = 2n.

Every face has 6 vertices, so every vertex must belong (on average) to 3 faces (it's the same argument as made before with edges). But, in a vertex, we can't have less than 3 faces, that is the same thing as saying: a vertex can't belong to less than 3 faces.

Thus, in every vertex, there are exactly 3 faces.

(Note: this is true for Szilassi's polyhedron, which is an example of a donut polyhedron with hexagonal faces)

We'll see now that, if the faces are convex hexagons, this is impossible!

The sum of the angles of a (convex) hexagon is 6x120°. Thus, each angle of each hexagon is, on average, 120°, thus, each vertex, on average, has a total of 3x120° = 360°. 

Here is the problem: if I have three faces that meet in a vertex and their angles are less than 180° (because the hexagons are convex), then the total sum of these angles can't be more or equal than 360°. This is true for every polyhedron you can think of; if you can imagine three faces that meet in a vertex, you can convince yourself; and one can prove it without too much trouble, but I will not do it here.

This means that in all the vertices, there are less than 360°, which is in contradiction with what was said before: on average, there are 360°. 

Having a contradiction, a donut polyhedron made only of convex hexagons (as the one in the picture) cannot exist...

 ❒

So, the picture found on the internet is trying to fool us: probably, the hexagons on the inside are not planar, but we can't see it from far away.

Let us return to our initial question: can we find a polyhedron made only of convex hexagons? The answer is no if it is a sphere (for Euler's formula) and no again if it is a donut, but maybe, adding holes to our donut... Maybe!

(Note: when I say "maybe", I really mean it! As far as I know, this is an open question in math: nobody knows the answer.)

Poliedri ed esagoni

[This post is in italian. Read here the English version]

I poliedri più conosciuti sono sicuramente quelli regolari, anche detti platonici: tetraedri, ottaedri, cubi, icosaedri e dodecaedri.

Sono fatti di triangoli, quadrati e pentagoni... Ma possiamo farne di esagoni?

La risposta è no, quantomeno se li vogliamo tutti uguali tra loro e regolari. Questo perché se affianchiamo tre esagoni questi riempiono tutto l'angolo di 360° (120° ciascuno) e non riusciamo a piegarli per creare qualcosa di tridimensionale. 

Anche i triangoli riempiono tutto l'angolo, ma per fare questo dobbiamo usarne sei. E quindi affiancandone solo tre, quattro o cinque riusciamo a creare poliedri: il tetraedro, l'ottaedro e l'icosaedro rispettivamente.

E se ci accontentiamo che solo alcune facce siano esagoni? In tal caso riusciamo a costruire poliedri. Ad esempio, usando anche pentagoni, otteniamo un icosaedro troncato, ovvero la forma del pallone da calcio.

Ma supponiamo di volere per forza che tutte le facce siano esagoni.

Magari basta prenderli non regolari? Ad esempio, di poliedri fatti di quadrati (cioè quadrilateri regolari) ne esiste solo uno: il cubo; ma se non chiedo che siano regolari ne esistono altri, come l'icosaedro rombico. Quindi, magari, di poliedri fatti di esagoni regolari non ne esistono, ma con esagoni irregolari sì.

La risposta, purtroppo, è sempre no!

Usando la formula di Eulero per i poliedri, che ci dice che 

vertici + facce = lati + 2 , 

(cioè il numero di vertici, sommato al numero di facce, è uguale al numero di lati più due) si dimostra infatti che non si riesce a costruire un poliedro fatto solo di esagoni, non importa quanto irregolari siano!

Questa è proprio una pessima notizia per chi ama i poliedri e gli esagoni :(

Ma non disperate! Non tutto è perduto!

La formula di Eulero, infatti, vale solo per i poliedri che sono "palle", cioè che non hanno buchi. Ma per i poliedri a forma di ciambella la formula di Eulero è diversa: 

vertici + facce = lati .

Possiamo quindi presentare un poliedro fatto tutto di esagoni! Signori e signore, ecco a voi... il poliedro di Szilassi:

Ha sette facce, tutte esagonali. Ogni faccia ha sei lati, e dunque tocca tutte le altre facce. Sei di queste facce sono esagoni concavi, uguali a due a due. L'ultima faccia è un esagono convesso, l'unico, e si trova "nel buco".

Ecco il suo sviluppo:

Purtroppo, qualcuno potrebbe obiettare che gli esagoni concavi sono brutti.

Io non sono d'accordo, ma per queste persone cercheremo di capire se è possibile trovare un poliedro con solo facce esagonali convesse.

Cercando su internet si può trovare questa immagine: un poliedro fatto a ciambella proprio come lo vogliamo noi.

Eppure... qualcosa non torna. Questo poliedro in realtà non può esistere! Ecco perché:

Diciamo che il nostro poliedro ha n facce esagonali.

I suoi lati quindi sono 3n, perché ogni faccia ha 6 lati, ma ogni lato appartiene a due facce.

Prendiamo ora la formula di Eulero per i poliedri a ciambella: vertici + facce = lati, quindi, sostituendo facce = n e lati = 3n, otteniamo vertici = 2n.

Poiché ogni faccia ha 6 vertici, questo significa che ogni vertice deve appartenere (in media) a 3 facce (stesso ragionamento che avevamo fatto per contare i lati). Ma in un vertice non possono incontrarsi meno di 3 facce, che è la stessa cosa che dire: un vertice non può appartenere a meno di 3 facce.

Quindi in ogni vertice si incontrano esattamente 3 facce.

(Nota: in effetti, questo è vero per il poliedro di Szilassi, che è un esempio di poliedro a ciambella con sole facce esagonali)

Vedremo ora che, se le facce sono esagoni convessi, questo è impossibile!

La somma degli angoli di un esagono (convesso) è 6x120°. Quindi ogni angolo di ognuno dei nostri esagoni misura in media 120°, quindi in ogni vertice, in media, ho un totale di 3x120° = 360°. 

Il problema è qui: se ho tre facce che si incontrano in un vertice, e i loro angoli sono minori di 180° (perché gli esagoni sono convessi), la somma totale degli angoli non può essere maggiore o uguale a  360°. Questo è vero per tutti i poliedri a cui puoi pensare; se riesci ad immaginare tre facce che si incontrano in un vertice puoi convincertene; e si può dimostrare abbastanza facilmente, anche se non lo farò qui.

Questo vuol dire che in tutti i vertici ho meno di 360° totali, che contraddice ciò che avevamo detto prima: in media ho 360° totali.

Poiché abbiamo una contraddizione, un poliedro a ciambella con tutti esagoni regolari (come quello nella figura) non può esistere. 

 ❒

Insomma, l'immagine trovata su internet ci sta imbrogliando: probabilmente gli esagoni al suo interno non sono planari, sono curvi, ma da lontano non si vede.

Torniamo quindi alla nostra domanda iniziale: possiamo trovare un poliedro fatto di soli esagoni convessi? La risposta è no se è una sfera (per la formula di Eulero) e ancora no se è una ciambella, ma magari, aggiungendo buchi alla nostra ciambella... chissà!

(Nota: quando dico "chissà", intendo davvero chissà! Che io sappia, questo è un problema aperto in matematica: nessuno ne conosce la risposta.)